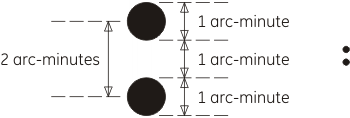
A person with 20/20 vision can distinguish a feature that is the size of one minute of arc. (There are 60 arc-minutes to a degree, and 60 arc-seconds in an arc-minute.) Since that's the smallest the eye can see, then a point, like a star, becomes a feature with a diameter of one minute of arc. In order for a person to distinguish two stars as separate, they need to see two points of light with a gap in between. So the stars need to be separated by 2 minutes of arc, center to center -- see the picture below.

Demonstrate this to yourself. Print this page (just the first page) and verify that each one of the two little dots in the picture above is 1/16 inch (1.5 mm) with a 1/16 inch gap between them. Stick the page up on the wall and stand back 18 feet (6 meters). If you have 20/20 vision you should *just* be able to resolve the two dots. At this distance, each dot is one arc-minute (60 arc-seconds) with a one arc-minute gap between them.
The Dawes Limit determines the smallest separation between two stars that the telescope can resolve, as described in the page on Resolving Power. Then for a person to see that separation, the telescope needs to magnify the separation to one the eye can resolve, which is 2 minutes of arc, or 120 arc-seconds. So then we have
Magnification × Resolving Power = 120 arc-seconds, and since resolving power is PR = 120/Do, then
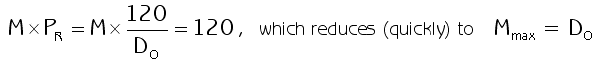
Wow. Do you see what this is saying? The maximum magnification of the telescope can be found by just looking at the diameter of the scope in mm. So if I look at the front of an 8-inch scope where it says "D=203mm", I know the maximum magnification is 203. I look at the front of my ETX where it says "D=90mm", I know the max magnification for my ETX is 90. I look at my Meade 6600 6-inch scope where it says "D=152mm" and I know the maximum magnification for that scope is 152.
This is very handy to know.
For more on the subject of matching eye to telescope, see the discussion
of optimum magnification on the
Surface Brightness page.
The eyepiece is equally easy to figure out. From the equation for
magnification we have
M = fO/fe, and we want the value of
fe-min to get us to Mmax = DO so then
DO = fO/fe-min, and therefore
fe-min=fO/DO. Since the f-ratio fR=fO/DO then we
have, quite simply, fe-min = fR Wow. Do you see what this is saying? The eyepiece focal length
to get the maximum magnification can be found by just looking at the
f-ratio for the scope! So if I look at the front of an 8-inch scope
where it says "f/10", I know the smallest eyepiece I can use with that
scope is a 10mm eyepiece. I look at the front of my ETX where it says
"f/13.8", I know the smallest eyepiece for my ETX is 14mm. I look at
my Meade 6600 6-inch scope where it says "f/5" and I know the smallest
eyepiece focal length to use with it is 5mm. This is also very handy to know. By the way you can confirm this for yourself... on a clear night,
looking at a bright star in your telescope, use an eyepiece with a
focal length in mm that matches the f-ratio and you will clearly see
the rings of the Airy disk,
telling you that you are operating at limit of the scope's resolution
(atmospheric conditions
permitting). If you compare manufacturer's specifications for maximum magnification
to the limit I give here, you'll find their number is often higher --
sometimes 2 or 3 times as high! Does this mean the manufacturers are
full of baloney? Well, actually, no. Well, some of them are, but for the most part, no.
It's true that once you get to the magnification Mmax =
DO, then going to higher magnification shows you no
additional detail, however -- sometimes making the image a little bit
bigger can make the detail a little easier to see. It really depends
on the image. For instance, in my ETX, with a calculated maximum magnification of 90,
I find that the binary star γ
(gamma) Leonis looks best at 90x, but the binary star Castor looks marginally
better at 140x. The separation's about the same, although Castor is quite
a bit brighter, so the dimming effect of higher magnification actually helps
to see the separation. When I look at Saturn, it looks a little bit better
at 140x (not entirely sure why), whereas Mars is definitely better at 90x.
Notice that in practice I'm willing to go to a magnification that's about
50% higher than the scope's maximum capability. That gives you an
image where objects are big with rounded edges (the Fisher-Price Toy image),
and beyond that you will likely find the image is just too blurry and
too dark. Depending on the author, there are several other formulas for maximum
magnification that you will see in other references, commonly either
50× scope diameter in inches (double what I've stated above) or
60× scope diameter in inches (2.4× what I conclude). I
encourage you to experiment and decide for yourself. I find that scope
diameter in millimeters is closer to reality (not to mention easier to
figure) than the other two very optimistic formulas. What happens if I just use the smallest eyepiece focal length I have
on hand? I've tried it... I can take my 90mm f/13.9 ETX and
put a 4mm eyepiece on it, which theoretically gives me a
magnification of DO
× fR ÷ fe = 90 × 13.9 ÷ 4 = 313.
When I look in the eyepiece I will notice two things: The reason things are blurry is because I have magnified the image so
much, I can see way beyond the limit that the scope can resolve. When
you expand the image so your eye can see details smaller than the
Dawes limit of the scope, you
now can see how the diffraction of light (or more likely the atmosphere)
blurs those tiny details.
The reason the image is so dark (I have a hard time telling that there
is an image) is because at this magnification, the scope is
operating at a surface brightness
of 0.2%, on a scale of 0 to 100%. That makes it two thousanths of
full brightness. It's a wonder I can see anything at all. When you reach the maximum magnification, your eye can now resolve
the Airy disk produced by
the telescope from the star's light. That means the stars are now
extended objects
subject to the dimming that occurs with magnification. Stars, which want to be points, don't like it when you turn them into
disks and spread their light out across the image. The faint ones
disappear pretty quickly as you increase magnification beyond the
Dawes Limit. On the other hand... this is precisely how I test the scope out when
I first get it... I use a ridiculously high power on a bright star
(straight up, on a crystal-clear night) and verify that I can see a nice,
tidy Airy disk, confirming that the scope is properly collimated. So
while you would not use a power way over the maximum magnification for
observing, it can be useful on occasion for testing the scope.
Your questions and comments regarding this page are welcome.
You can e-mail Randy Culp for inquiries,
suggestions, new ideas or just to chat. Finding the Eyepiece for Maximum Magnification
How Maximum is Maximum?
Practice
So How About If I Just Go To the Highest Possible Magnfication?
Yes but... where did the stars go?
 Back to Telescope Equations Home Page
Back to Telescope Equations Home Page
Questions
Updated 11 May 2019